The variable Reasoning, in all of the equations, generally acts to hold an individual’s Attention level constant. Depending on whether or not the Reasoning is used to hold both Inattention to Threat and Inattention to Efficacy constant, to hold Inattention to one constant and not the other, or to not hold Inattention on either constant, an individual’s valuation of an entity may remain constant, elevate toward positive infinity, or descend toward its existential value of positive one for forms One and Two of the Avoidance of Pain Equation. For form three, as opposed to descending toward its existential value of positive one, the Inattention devoted to a feature will generally decrease unless Inattention to it is actively maintained. However, if the Inattention level is already below normal (meaning the entity is being given a heavy amount of attention), then inattention will increase and balance out to the Inattention level devoted to everything else unless it is actively maintained.
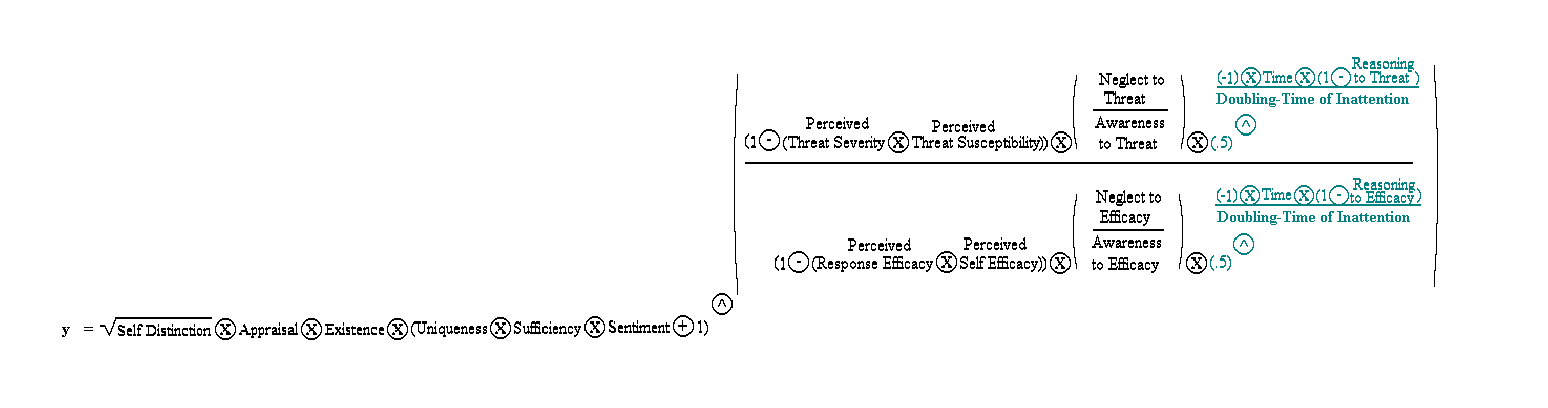
Of note, the expression for the Doubling-Time of Inattention has a coefficient of negative one (-1), which further distinguishes it from the expression for the Half-Life of Attention. Whereas the Doubling-Time of Inattention is the time it takes the Inattention level to double its initial value, the Root-Life of Inattention is a novel concept that measures something else. The Root-Life of Inattention represents the time it would take Inattention (if defined as a ratio of Neglect to Awareness) to revert back toward one by a factor of one square root. Form three does not require the coefficient of negative one (-1) in the expression. Similar to form three of the Avoidance of Pain equation, the Inattention level is taken to the power of one-half (.5) for form three of the Pursuit of Pleasure equation.
As the x-variable, representing Time, is now officially implemented into the equation, Category I, II, III, and IV Emotions can now be modeled: Categories of Emotions.
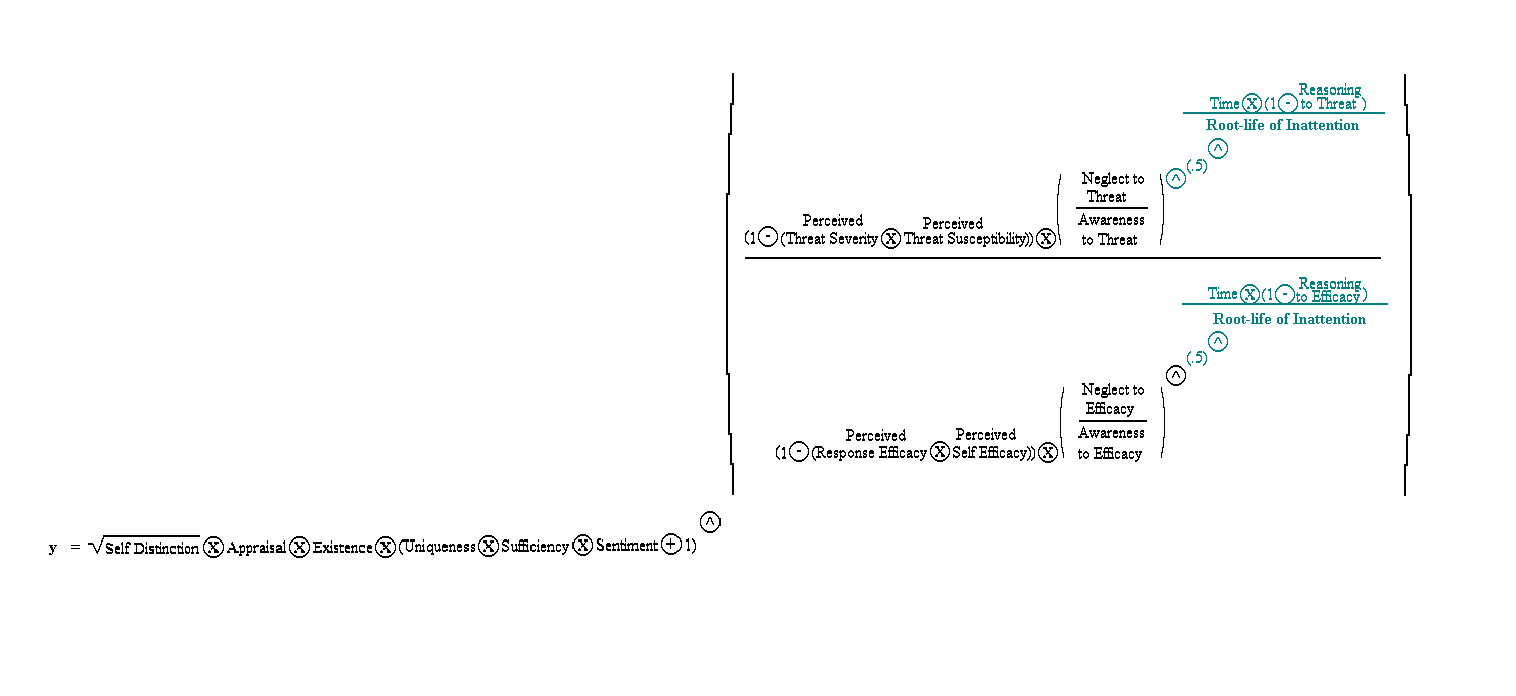
Form One: Pursuit of Pleasure Equation with Exponential Decay and No Error for the Half-Life of Attention (Continued)
Form One: Pursuit of Pleasure Equation with Exponential Decay and No Error for the Half-Life of Attention
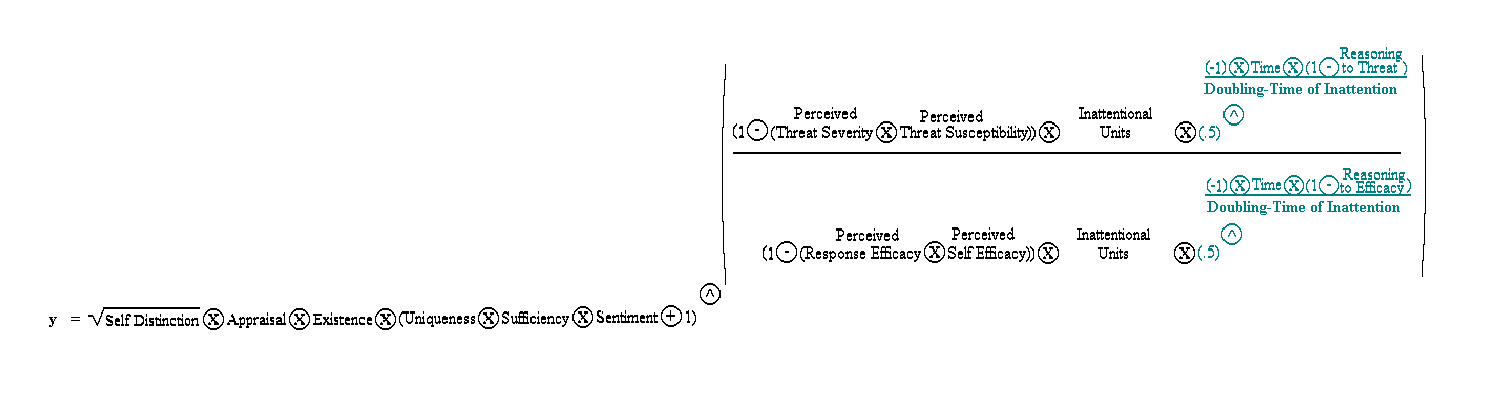
Form Two: Pursuit of Pleasure Equation with Exponential Decay and Error for the Half-Life of Attention (Continued)
Form Two: Pursuit of Pleasure Equation with Exponential Decay and Error for the Half-Life of Attention
Form Three: Pursuit of Pleasure Equation with Valuation Resilience
Form Three: Pursuit of Pleasure Equation with Valuation Resilience